

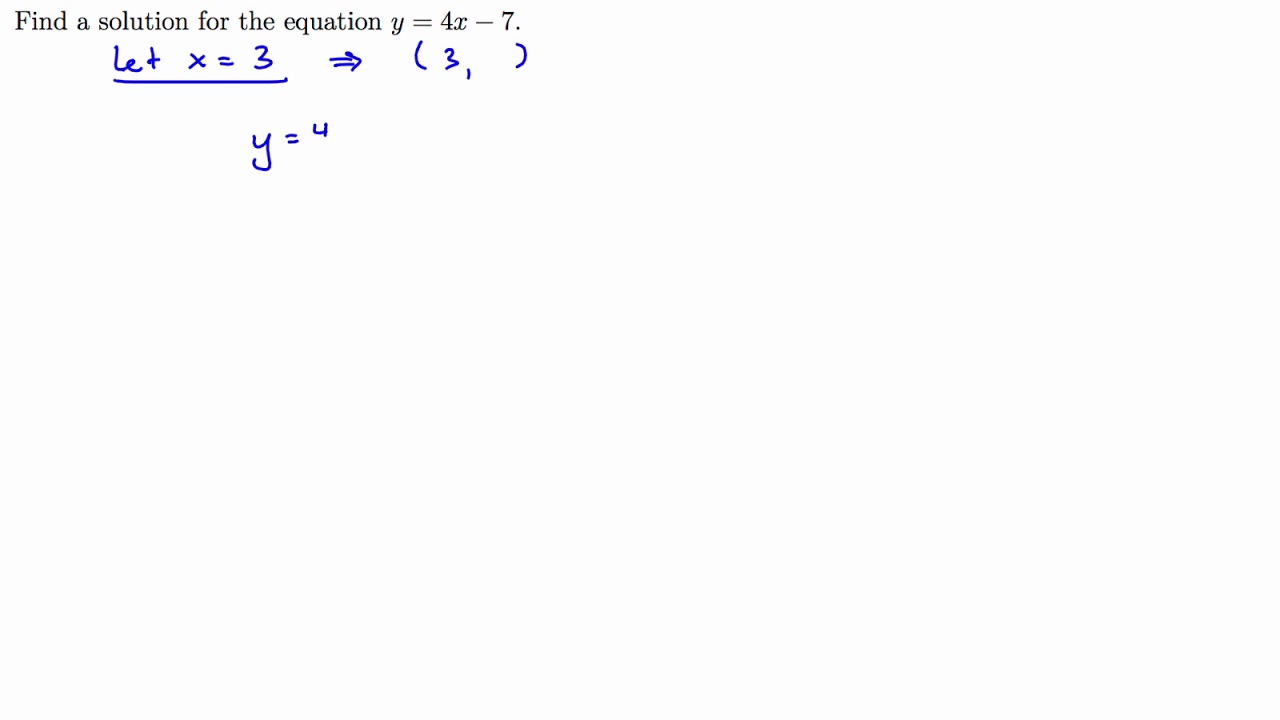
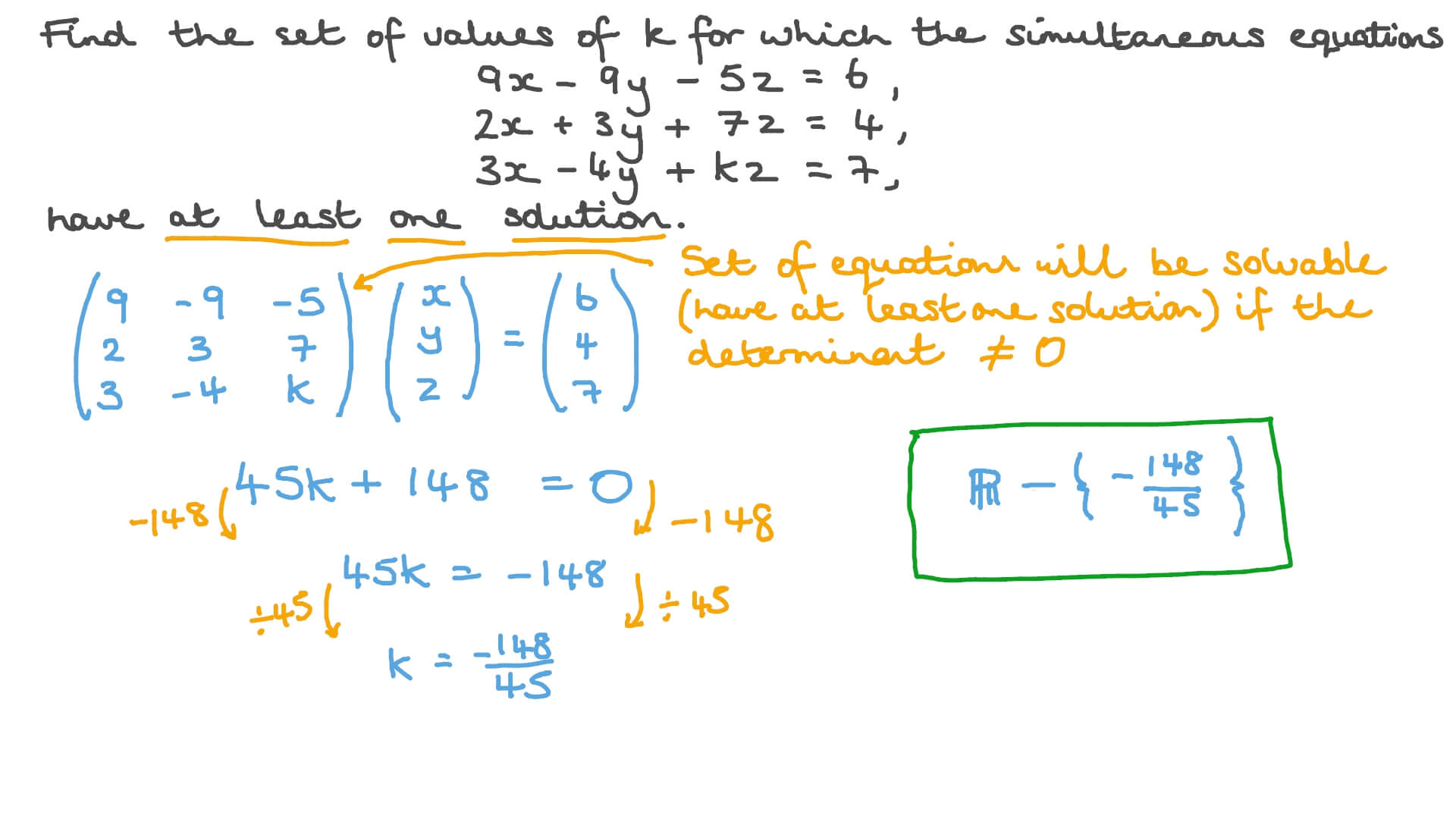
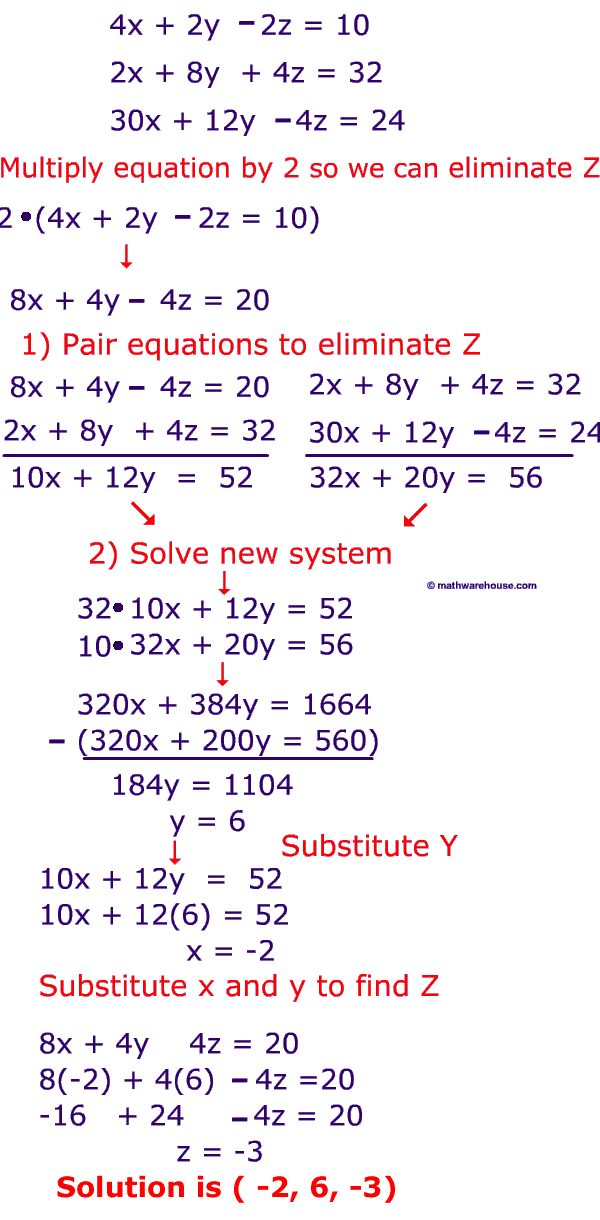
While you could multiply the second equation by 25 to eliminate L, the numbers will stay nicer if you divide the first equation by 25. To make things easier, rewrite the equations to be in the same format, with all variables on the left side of the equal sign and only a constant number on the right. She sells twice as many medium photos as large photos. The number of small photos is the same as the total of medium and large photos. The total of her sales must be $300 to pay for the booth. In this case the unknown values are the number of small, medium, and large photos. To set up the system, first choose the variables. If her sales go as usual, how many of each size photo must she sell to pay for the booth? She also sells twice as many medium photos as large. She usually sells as many small photos as medium and large photos combined. She prices the photos according to size: small photos cost $10, medium photos cost $15, and large photos cost $40. Step 6: Use the two found values and one of the original equations that had all three variables to solve for the third variable.Īs with systems of two equations with two variables, you may need to add the opposite of one of the equations or even multiply one of the equations before adding in order to eliminate one of the variables.Īndrea sells photographs at art fairs. Step 5: Use that value and one of the equations from the system in step 3 that involves just two variables, one of which was g that you already know. Step 4: Solve the resulting equation for the remaining variable. These equations can be added to eliminate f. Step 2: The third equation has no h variable, so there’s nothing to eliminate! You have a system of two equations and two variables. The first two equations can be added to eliminate h.
Step 1: Choose two equations and eliminate a variable. Check your answer in all three equations ! Do this by using one of the original equations and the values of the found variables from steps 4 and 5.ħ.
Do this by using one of the resulting equations from steps 1 and 2 and the value of the found variable from step 4.Ħ. Solve the final equation for the remaining variable.ĥ. Use the resulting pair of equations from steps 1 and 2 to eliminate one of the two remaining variables.Ĥ. Choose another pair of equations and use them to eliminate the same variable.ģ. Choose two equations and use them to eliminate one variable.Ģ.
Just as two values can be written as an ordered pair, three values can be written as an ordered triplet: ( x, y, z) = (1, 2, 3).ġ. Since x = 1, y = 2, and z = 3 is a solution for all three equations, it’s the solution for the system of equations. There are three variables and three equations. This is the case that you are usually most interested in. Let’s start by looking at Case 1, where the system has a unique (one) solution. And this can also occur when the three equations graph as the same plane. This occurs when the three planes intersect in a line. (Note that two of the equations may have points in common with each other, but not all three.) Below are examples of some of the ways this can happen.Ĭase 3: There are an infinite number of solutions. The three planes do not have any points in common. In order for three equations with three variables to have one solution, the planes must intersect in a single point.Ĭase 2: There is no solution. Let’s look at this visually, although you will not be graphing these equations.Ĭase 1: There is one solution. Just as when solving a system of two equations, there are three possible outcomes for the solution of a system of three variables.


 0 kommentar(er)
0 kommentar(er)
